

The paper entitled “Probabilistic Modelling using Monte Carlo Simulation for Incorporating Uncertainty in Least Cost Path Results: a Roman Road Case Study” [1] submitted by J. Lewis presents an innovative approach to applying Least Cost Path (LCP) analysis to incorporate uncertainty of the Digital Elevation Model used as the topographic surface on which the path is calculated.
The proposition of using Monte Carlo simulations to produce numerous LCP, each with a slightly different DEM included in the error range of the model, allows one to strengthen the method by proposing a probabilistic LCP rather than a single and arbitrary one which does not take into account the uncertainty of the topographic reconstruction. This new method is integrated in the R package leastcostpath [2].
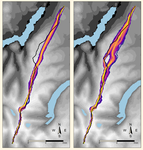
The author tests the method using a Roman road built along a ridge in Cumbria, England. The integration of the uncertainty of the DEM, thanks to Monte Carlo simulations, shows that two paths could have the same probability to be the real LCP. One of them is indeed the path that the Roman road took. In particular, it is one of two possibilities of LCP in the south to north direction.
This new probabilistic method therefore strengthens the reconstruction of past pathways, while also allowing new hypotheses to be tested, and, in this case study, to suggest that the northern part of the Roman road’s location was selected to help the northward movements.
[1] Lewis, J., 2020. Probabilistic Modelling using Monte Carlo Simulation for Incorporating Uncertainty in Least Cost Path Results: a Roman Road Case Study. SocArXiv, mxas2, ver 17 peer-reviewed and recommended by PCI Archaeology, 10.31235/osf.io/mxas2.
[2] Lewis, J., 2020. leastcostpath: Modelling Pathways and Movement Potential Within a Landscape. R package. Version 1.7.4.
After reviewing the edited version of the manuscript, I can confirm that the author has taken into account all the comments made. I therefore recommend the preprint for publication.
https://doi.org/10.24072/pci.archaeo.100005.rev21DOI or URL of the preprint: https://doi.org/10.31235/osf.io/mxas2
Dear all,
Firstly, thank you for reviewing the manuscript. The comments were extremely constructive and provided a lot to think about. I have taken the feedback on board and believe that it is now a clearer and stronger article.
Please see my rely to the Reviewer’s comments attached.
For reference, the updated paper has been uploaded to SocArXiv (Version 17) (https://osf.io/preprints/socarxiv/mxas2) and the updated data/code is available at: https://doi.org/10.5281/zenodo.4074956
I look forward to hearing your thoughts.
Kind regards, Joseph Lewis
In accordance with the reviews and recommendations of the peer reviewers, I recommend that the paper be revised by the author. For details, please see the peer reviews.
Probabilistic Modelling using Monte Carlo Simulation for Incorporating Uncertainty in Least Cost Path Results: a Roman Road Case Study. (DOI https://doi.org/10.31235/osf.io/mxas2)
This paper discusses the use of the Monte Carlo simulation for producing a probabilistic assessment of the accuracy of Least Cost path modelling. The author’s approach is based on generating 1000 realizations of random error fields representing the vertical error of the DEM (drawn from RMSE) and by spatially propagating this error throughout the DEM. This process is integrated into the R package leastcostpath for LCP modelling. Leastcostpath is the second designed R package of its type dealing with least Cost path modelling following Movecost (Alberti, 2019). The latter calculates accumulated slope-dependent anisotropic cost-surfaces and least-cost paths.
This paper offers an innovative approach to LCP analysis by modelling DEM uncertainty. It is well structured from a methodological and theoretical perspective. The abstract adequately reflects the content of the paper. The research question is clearly defined. The aims are stated and their significance is clearly explained. I therefore recommend that this paper be accepted. The following comments aim at providing recommendations for refining the preprint.
The Monte Carlo simulation requires substantial computational capacities for error propagation if the input DEM has a high spatial resolution (Gesch et al., 2020; Temme, Heuvelink, Schoorl, & Claessens, 2009) or when different cost functions are used comparatively for modelling the LCP. The number of modelled scenarios to be computed will moreover be increased if other factors, such as incorporating barriers with various impedance values, are included in modelling movement.
The following reference (Verhagen, Nuninger, & Groenhuijzen, 2019) could be added in the paragraph extending from lines 22-33 when discussing the role of the DEM, the slope and the key factors in LCP modelling.
Lines 60-63: It is worth highlighting that the Monte Carlo Simulation could also be applied to DEM with high accuracy and high spatial resolution. See for instance the study conducted by Gesch et al. (2020). As has been stated by Temme et al. (2009), “A high-resolution DEM may still have a greater uncertainty than a low resolution DEM if we are less certain of its attribute values”.
A study conducted by Herzog & Yépez (2015) on the impact of DEM on archaeological GIS studies could be added in lines 64-71, where the author highlights the rarity of archaeological studies discussing the vertical error and its impact on LCP modelling.
Lines 96-97: Perhaps it would also be useful to explain in a few sentences the concept of spatial autocorrelation (see Temme et al., 2009) as it could help provide a better understanding of the usefulness of the neighborhood autocorrelation filter method.
Lines 203-205: It would be better to evaluate the RMSE of the SRTM DEM of the study area based on a number of reference points (datum points) rather than relying on consensus view of a minimum RMSE value equal to 9.73 m worldwide.
Lines 227-229: The author states that: “Effective slope, which takes into account the direction of descent, was computed in leastcostpath by calculating the difference in elevation between cells and their sixteen neighboring cells”. Is this based on the effective slope equation developed by Yu, Lee, & Munro-Stasiuk (2003)? In this case, the distance between cell centers should be also taken into account in the calculation of the slope value (See also Herzog 2014).
Lines 239-240: It is stated that the maximum distance from the known location of the High Street Roman road to the computed LCP from the south-to-north is 85% less than the maximum distance of the LCP calculated from north-to-south. In lines 292-294 the author also notes that the LCP from north-to-south is less accurate with 85% greater maximum distance than the LCP from south-to-north. Would it be possible to clarify how this percentage was calculated? Based on the numbers exposed in table 1 the maximum distance from the Least Cost Path calculated from North to South to the known location of the High Street Roman road of is 825.93 m while the maximum distance from the Least Cost Path calculated from South to North is 332.97 m. Thus, the percentage decrease from 825.93 to 332.97 is 59.68% while the percentage increase from 332.97 to 825.93 is 148.04%.
It would be preferable to add a scale and a north arrow to figures 6-8 since the author designates the computed LCP according to cardinal directions.
References:
Alberti, G. (2019). movecost: An R package for calculating accumulated slope-dependent anisotropic cost-surfaces and least-cost paths. SoftwareX, 10, 100331. doi: https://doi.org/10.1016/j.softx.2019.100331.
Gesch, D., Palaseanu-Lovejoy, M., Danielson, J., Fletcher, C., Kottermair, M., Barbee, M., & Jalandoni, A. (2020). Inundation Exposure Assessment for Majuro Atoll, Republic of the Marshall Islands Using A High-Accuracy Digital Elevation Model. Remote Sensing, 12(1). doi: 10.3390/rs12010154.
Herzog, I., (2014). Least-cost Paths – Some Methodological Issues. IA. https://doi.org/10.11141/ia.36.5 461.
Herzog, I., & Yépez, A. (2015). The impact of the DEM on archaeological GIS studies: A case study in Ecudaor Paper presented at the Conference on Cultural Heritage and New Technologies, Vienna.
Temme, A. J. A. M., Heuvelink, G. B. M., Schoorl, J. M., & Claessens, L. (2009). Chapter 5 Geostatistical Simulation and Error Propagation in Geomorphometry. In T. Hengl & H. I. Reuter (Eds.), Developments in Soil Science (Vol. 33, pp. 121-140): Elsevier.
Verhagen, P., Nuninger, L., & Groenhuijzen, M. R. (2019). Modelling of Pathways and Movement Networks in Archaeology: An Overview of Current Approaches. In P. Verhagen, J. Joyce & M. R. Groenhuijzen (Eds.), Finding the Limits of the Limes: Modelling Demography, Economy and Transport on the Edge of the Roman Empire (pp. 217-249). Cham: Springer International Publishing.
Yu, C., Lee, J. A. Y., & Munro-Stasiuk, M. J. (2003). Research Article: Extensions to least-cost path algorithms for roadway planning. International Journal of Geographical Information Science, 17(4), 361-376. doi: 10.1080/1365881031000072645.
https://doi.org/10.24072/pci.archaeo.100056.rev11This well-organised and focused study presents a simple methodological improvement to LCP analysis, one of the mainstays of computational landscape archaeology. LCP is not an ideal method for landscape archaeology, and it is not possible to truly conduct a LCP analysis probabilistically, as there is by definition only one least cost path. Moreover, it is difficult to discern how this article can affect the application of LCP in archaeological interpretation.
Still, it is an often used technique, and the author’s attempt to bring uncertainty into LCP deserves recommendation. The authors wish to take into account the uncertainty raised by elevation error, a common feature of all DEM datasets used in LCP analysis. This form of error has not be handled systematically in the past, and threatens the integrity and applicability of LCP analysis. As such, the article is poised to make an important contribution to one of the most widely-used techniques in computational archaeology.
The author’s approach involves using Monte Carlo simulation to take into account all of the possible elevations on a given path in an archaeological dataset. This allows the calculation of a range of possible distances, which can be visualised as a probability cloud, rather than compute the stated elevations along a single path. This provides a range of likely paths, and can be used to visualize the most likely vector of movement across a DEM in a particular direction. A visualisation of the averaged paths can be found on Line 284.
I would come back around to my caveat, however. The approach the authors have outlined can be used to identify a probabilistic range of results. It would be useful if the author illustrated how this probabilistic range of results can shape archaeological interpretation. How does realizing there is a range of possible distances affect our understanding of the Roman Road network? What does a 400-meter difference mean from an interpretative standpoint? What do these probabilistic thresholds mean for the interpretation of LCP results?
https://doi.org/10.24072/pci.archaeo.100056.rev12